

|
||
|
|
||
The Torsional (-Flexural) Buckling Check is executed according to EN 1993-1-3 art. 6.2.3 and EN 1993-1-1 art. 6.3.1.4.
The buckling curve for torsional (-flexural) buckling is taken as the z-z buckling curve according to the table given in "Flexural Buckling".
The value of the elastic critical load Ncr is taken as the smallest of Ncr,T (Torsional buckling) and Ncr,TF (Torsional-Flexural buckling).
The elastic critical load Ncr,T for torsional buckling is calculated according to Ref.[17].

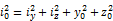
With
|
E |
Modulus of Young |
|
G |
Shear modulus |
|
It |
Torsion constant |
|
Iw |
Warping constant |
|
lT |
Buckling length for the torsional buckling mode |
|
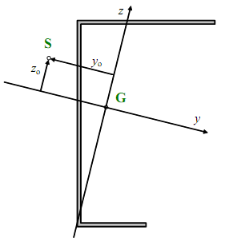
y0 and z0 |
Coordinates of the shear center with respect to the centroid |
|
iy |
radius of gyration about the strong axis |
|
iz |
radius of gyration about the weak axis |
The elastic critical load Ncr,TF for torsional flexural buckling is calculated according to Ref.[17].
Ncr,TF is taken as the smallest root of the following cubic equation in N:
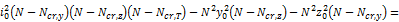 0
0
With
|
Ncr,y |
Critical axial load for flexural buckling about the y-y axis |
|
Ncr,z |
Critical axial load for flexural buckling about the z-z axis |
|
Ncr,T |
Critical axial load for torsional buckling |
In case a diaphragm is used, independent on which side, the augmented It will be used also in Torsional Buckling.
For more information on diaphragms see "Annex D: Use of diaphragms ".